DOUGLAS A. PAINE
WILLIAM L. PENSINGER

(Suggestion for McIntosh users: Using Virtual PC
with IBM-platform browsers will insure
text-embedded math symbols are read properly.)

TABLE OF CONTENTS
Abstract
Introduction
1.	The Thermodynamic Superconductant Model
2.	Interpretation
3.	The Resultant Limited Atmospheric Domain Wave Equations
4.	Establishing a Test Case
5.	General Relativity and the Limited Atmospheric Domain
6.	The Relativism of Absolute Limits
7.	An Initial Look at the Superconductant Role of Nonequilibrium 
	    Negentropic Processes
8.	Defining the Quantum State within the Limited Atmospheric
	    Domain
		a. Planck's Law
		b. The Relativistic-Quantum Definition of Temperature
9.	On the Relativistic-Quantum Domain
	    and Nonequilibrium Themodynamics
10.	Summary and Conclusions
Acknowledgments
11.	References
12.	Appendices

Introduction

In order to theoretically describe superconductant processes within an atmospheric domain, this paper will adopt the hierarchical methodology proposed by Bohm and Hiley (1975). Section 1 will detail the superconductant description, wherein a supersystem-system-subsystem composite is envisioned as consisting of:

1. the total number of air parcels comprising a given limited atmospheric domain structure (supersystem);

2. an individual air parcel (system, or localized domain structure);

3. the subsystem of gaseous molecules comprising the air parcel.

In this microscopic approach, the air parcel is hypothesized to assume the role of an elemental oscillator whose function is to communicate the quantum potential within the atmospheric domain. The primary variables descriptive of the stable and unstable states within this closed dynamic consist of the reference level and critical temperatures, respectively.

In Section 3, consisting of the theoretical hydrodynamic description, the supersystem-system-subsystem methodology is shifted to encompass:

1. the embedding domain (as supersystem) surrounding a given limited atmospheric domain structure;

2. the total number of air parcels comprising the limited atmospheric domain structure (system);

3. the individual air parcel (as subsystem).

From the point of view of this macroscopic approach, a coherent wave phenomenon is proposed which maintains the conservation of angular momentum within, as well as communicating the quantum signal beyond, the limited atmospheric domain. This description displays some of the essential features of both quantum and relativistic physics. The fundamental frequency of the oscillating parcel is found to function as an ordering principle by establishing the limits of the spatial domain.

The remaining sections of the paper will provide empirical evidence gathered to support the hypothesis that relativistic physics and quantum nonlocality are central themes governing dynamic regimes on all scale levels. We will specifically investigate which of the prevailing assumptions within the classical interpretation of relativity and quantum theory must be altered to provide the bridgework capable of extending these ideas across a broader range of physical spectra. It appears that new concepts of space will arise as we begin explorations into the ordering functions of time.

1. The Thermodynamic Superconductant Model

We will begin by relating atmospheric superconductivity to two variables:

T_o, the reference level temperature which a limited atmospheric domain maintains when it is nonaccelerative;

T_c, the critical temperature which the same limited domain attains when it becomes fully accelerative due to radiation exchange.

Both temperatures within the Earth’s lower atmosphere often lie within the range of 270K to 330K, or well above absolute zero.

Consider the nonaccelerative state of the totality of air parcels comprising a limited atmospheric domain (supersystem) to be the stable state. Consider the transition towards the fully accelerative state to be a transition towards an unstable state. What effect does radiation impinging upon a limited atmospheric domain have with regard to the hypothesized transition between the stable and unstable states?

Let us define the stable environment of the total number of air parcels, which constitutes the supersystem associated with the given limited atmospheric domain, to be representative of hydrostatic equilibrium. The resultant bouyant force at any level is zero

where the inverse distribution of air parcel density in the environment (r_e) multiplied by the pressure gradient between levels is exactly counterbalanced by g, the gravitational acceleration. Let us assume that a given parcel always adjusts its pressure to the pressure of the environment. The following equation describes the time rate of change of parcel motion

where r_p designates the parcel’s perturbation density.

Eliminating ¶P/¶z from (1) and (2)

Utilizing the equation of state (P = rRT) for the parcel and the environment respectively, (3) is rewritten

where T_e and T_p refer to the environmental and parcel temperatures, respectively. (Later, we will find it instructive to substitute T_e = T_o as descriptive of an environmental reference temperature, where T_o £ T_p £ T_c.

At this point, we wish to consider how a net radiation exchange between the parcel (system) and its environment (supersystem) influences the vertical motion () of a given elemental parcel. In general, the radiative flux for a blackbody is given by

The reader will note that we are taking the unusual step of defining a thermodynamic concept (Stefan-Boltzman Law) at the system level: the air parcel, composed of a subsystem of gaseous molecules, does not call to mind the traditional interaction of molecules constituting a liquid. However, this step, invoking a superfluid approach to atmospheric dynamics, will directly link the thermal exchange of energy achieved between the localized domain structure (parcel) and the limited atmospheric domain exhibiting quantum properties. We are thus hypothesizing that blackbody physics must be an integral part of all superconductant processes. We, therefore, seek to mathematically describe the manner in which the quantized signal, or time of transition from T_o to T_c, is communicated from subsystem to system to supersystem. To capture the essence of superconductance, we, therefore, assume that spontaneous localization at any one of these three levels is synchronously tied to spontaneous fusion of the signal into the next higher level. This hypothesis as to the function of time ordering in the interplay between the subsystem (molecular), the system (air parcel), and the supersystem (limited atmospheric domain) will lend itself to several empircal tests to be established beginning with Section 4.

For an idealized spherical parcel, the net radiation flux is

and this expression is equivalent to the total change in energy per unit time of the parcel due to radiational processes.

Solving for

The density in (8) is to be considered a kind of mean density. The constant C_p refers to the specific heat at constant pressure, and s is the Stefen-Boltzmann constant.

Since r_p = f (P_e, T_p) and

we solve for r_p, the radius of the spherical parcel,

Substituting (9) into (8)

After the use of a Taylor series expansion and an order of magnitude argument outlined in Appendix A, we obtain

Despite the apparent complexity of expression (11) for the time rate of temperature change of the oscillating parcel due to radiation impingement, it is well to recall that the righthandside of (11) is merely a function of z, the position of the parcel relative to a reference level defined by T_e = T_o.

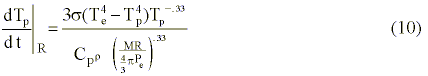
In this regard, we must allow the parcel to change its temperature when moving back and forth to and from the reference state (T_p = T_o). This temperature change due to vertical motion involves the adiabatic lapse rate g_d:

Thus, the total temperature change of the parcel is

where

We now have two differential equations, (4) and (13):

Observing that,

where g is the actual temperature lapse rate through the environment, we obtain from (14a) and (14b)

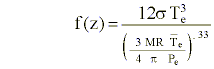
Let t = (T_e-T_p), and after differentiating (16) once with respect to time, we have

This equation has the form

if S(x) = 0 and the functions P, Q, and R reduce to constants.

Equations (17) and (18) describe a harmonic oscillator. If the coefficient of t in term 3 is positive, the solution for t is a sinusoidal function of time. That is, the parcel will oscillate about its original position with a fundamental frequency given by:

In the stable case, this may be verified by substitution for t into Equation (17)

where A and B are constants of integration. The inverse of n_o defines the minimum time for the process of spontaneous localization to occur at the system level.

2. Interpretation

By substituting (T_o-T_c) for (T_e-T_p) in Equation (17), we obtain a dynamic description for an air parcel which is envisioned to oscillate between the reference level temperature (T_o) and the critical temperature (T_c) uniquely established for a given limited atmospheric domain. Term 3 on the lefthandside of (17) appears in the role of a work term:

wherein the expansion-contraction of the parcel as it traverses T_o«T_c represents a means of information exchange within the limited atmospheric domain. This is a type of carrier function which contributes no component to the actual information exchanged, but does define the extent of this domain, as will be shown in Section 4. In this manner, an active temporal parameter partitions the spatial reference frame, or, in other words, the fundamental frequency of the oscillating parcel functions as an ordering principle by establishing limits of the involved spatial domain.
Term 2:

represents exchange of information within the limited atmospheric domain by describing changes of the parcel’s periodicity. Thus, the time rate of change of t exists as a central component for information exchange which may be empirically determined for a given limited atmospheric domain. Finally, the second-order time rate of change of t, expressed in term 1 of Equation (17) as

maps the limited atmospheric domain’s exchange of information between itself and other (embedding) domains.

3. The Resultant Limited Atmospheric Domain Wave Equations

The supersystem-system-subsystem methodology will now be shifted to encompass the embedding environment, the limited atmospheric (embedded) domain, and the air parcel, respectively. By making the limited atmospheric domain the localized domain structure (system), and the air parcel the subsystem, we will be able to construct a set of wave equations descriptive of the postulated limited atmospheric domain’s information exchange process.

As mentioned earlier, we associate the balance of forces upon the air parcel described in Equation (1) to be descriptive of a hydrostatic (stable) state. A net imbalance of forces and consequent acceleration of the parcel described in Equation (3) is descriptive of the nonhydrostatic (unstable) state. In order to codify the transitions of the limited atmospheric domain represented by a group of air parcels transiting between the hydrostatic and nonhydrostatic states, we first identify the two velocity vectors:

chosen for their representation of the nonaccelerative and accelerative states, respectively. By further defining a first-order spacetime del operator:

and a second-order spacetime del operator:

we will be able to map the first- and second-order time rates of change of the two vector quantities as two aspects of a coherent wave phenomenon.

To do this, we first equate the spatial curl of each vectorial quantity to the time rate of change of the opposing vectorial quantity (see Appendix B):

Note that “time” as invoked in these equations, rather than appearing as a passive backdrop to the ensuing dynamics, is now central and an active participant in the dynamic processes by which information is exchanged within the superconductant, limited atmospheric domain. The relevant time has been previously established in Equation (19) as the fundamental period (n_o^-1) of the air parcel, which describes the minimum time for spontaneous localization to occur. The macroscopic view afforded by these wave equations simply summarizes the (microscopic) view derived from the description of a single parcel governed by the middle term in Equation (17). As an ensemble of parcels accelerate toward the limiting phase velocity (±c, units in cm s^-1) descriptive of the macroscopic wave, the parcels alternate between being participants in the full field intensities of the hydrostatic
(T_p = T_o) and nonhydrostatic (T_p = T_c) states. In this manner, the wave phase velocity mediates the transition between maximized field strengths by imposing a limiting velocity constraint upon individual air parcels, thus subscribing to principles of the Special Theory of Relativity. In the hydrodynamics terminology of atmospheric physics, these equations allow us to record the change of parcel spin, within the limited atmospheric domain, between the vertical () and horizontal components () of relative vorticity (s^-1).

If we next invoke the second-order spacetime del operator in order to describe the aspect of the wave phenomenon associated with the first term on the lefthandside of Equation (17):

we find that the air parcels transit toward a limiting acceleration governed by the phase acceleration (±c', units in cm s^-2) of the macroscopic wave. We recall that as the phase acceleration mediates the parcel’s second-order time rate of change between T_o«T_c, it manifests the exchange of information to an from the limited domain.

Definition of a wave phase acceleration, a natural extension to that of a wave phase velocity often used in physical problem solving, becomes necessary in order to rigorously codify this exchange of information between the embedded and embedding domains. The subsequent exchange of relative vorticity (or angular momentum) within the limited atmospheric domain (Equations 21a and 21b), as well as between the embedded and embedding domains (Equations 22a and 22b), will be documented beginning in Section 5. It is observed that the notion of a limiting acceleration is a step beyond traditional Special Relativity, a step necessitated by existence of multiple limited atmospheric domains established by fundamental frequencies of oscillating air parcels.

4. Establishing a Test Case

Taking our cue from the work term identified in Equation (17), we will establish a limited atmospheric domain for testing our concepts by considering two criteria:

1. the regime must have a well defined variance away from the standard atmospheric lapse rate;

2. the regime must have an equally well defined change between an initial reference level temperature and a final CRITICAL temperature.

Both criteria are met by considering a limited domain which transits from an initial mean potential temperature () to a final CRITICAL temperature designated by the domain’s mean equivalent potential temperature (). [[The potential temperature was defined by Poisson as the temperature obtained by an air parcel brought adiabatically to 1000 millibars, whereas q_E includes the diabatic addition of heat realized by condensing all of the parcel’s available moisture before its arrival at 1000 mb. Again, we are purposely extending to the supersystem level concepts which are commonly confined to individual parcels at the system level.]] This methodology is appealing because meteorology frequently defines the (hydro)static stability of the atmosphere in terms of standard or ideal (potential) temperature variation in the vertical, versus the nonhydrostatic (or convective) stability defined in terms of the vertical variation of q_E.

Upon forming an inverse ratio between the three-dimensional variations of these two stabilities (Sanders and Olson, 1967):

where X, Y, and Z are scale factors and f is the Coriolis parameter, we are able to describe the observed atmosphere’s degree of gravitational damping versus that imposed by the variations of stability found within the standard atmosphere (Kaplan and Paine, 1972). The resultant ratios displayed in Figure 1a delineate the horizontal extent of a limited atmospheric domain contained within the 2.6 isopleth. Our test case, having met the above criteria, is situated over the Great Lakes region. The test period covers a 6 h time span, ending 0000 Greenwich Mean Time (GMT) 5 November 1967. The vertical extent of this limited domain is shown in Figure 1b: the 0000 GMT vertical cross-section indicates the lower boundary of a strong inversion found to slope downward from 3 km to 2 km, some 400 km to the north and south of Lake Erie. The abrupt change of stability within this layer (representing a temperature increase with respect to height) forms the upper boundary of the limited domain, while the Earth’s surface comprises its lower boundary.

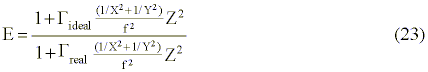
We see from Figure 1a that maximum deformation in the ratio formed between the hydrostatic and nonhydrostatic stabilities is situated at the 4.1 isopleth analyzed over southwestern Lake Erie. Beginning shortly after 1800 GMT 4 November, radar reports indicated that intensifying convection moved rapidly eastsoutheastward at
30 m s^-1 from this region. Figure 2 shows a mesolow (L) 5 h later, which had developed below the rotating cluster of squalls, preceded by an anomalous pressure increase of +7 mb when contrasted to the prevailing macroscale surface pressure pattern. The surface streamline pattern, drawn from the observed wind field, shows a mesocyclonic circulation located beneath the rotating convective strom, preceded by an anti-cyclonic outflow point. Surface divergence values diagnosed upon a 42 km gridlength equalled +10^-4 s^-1 over the clockwise swirl. Convergence (stipple pattern) on the order of -10^-4 s^-1 and a cyclonic vorticity maximum were found centered over the mesolow.

With snowfalls of up to 50 cm left in the wake of this intense convective storm, we may assume that there was an efficient flux of sensible and latent heat from the relatively warm (+1°C) water of Lake Erie entering into the lower atmosphere. A numerical simulation of the event (Kaplan and Paine, 1973) was able to capture the subsequent rapid change in the lower atmospheric temperature field, as shown in Figures 3a and 3b. Maximum temperature increases of +7°C (6 h)^-1 were coincident with the predicted maximum snowfall.

A quantitative measure of the radiation (q) from Lake Erie is obtained by using Equation (24)

where the diabatic heating (Dq) for the 6 h period was set to +7K. The height of the convective column (Dz) was determined using the 4.5 km cloud top as recorded over Lake Erie by Detroit 10-cm radar. The average temperature within the heated column equalled 263K, and the mean potential temperature was 280K, as measured by actural radiosonde data. Utilizing these values plus the appropriate mean density of the column and C_p, we found q expressed in erg cm^-2. After converting to calories and dividing through by time to obtain the diabatic heating rate, we obtained 1.96 cal cm^-2 min^-1, an unusually high value which is nearly equal to the solar radiation parameter.

To complete our brief thermodynamic description of the problem, it is essential that we solve Equation (19). The fundamental frequency for this limited atmospheric domain was found to be:

n_o = 1.64 x 10^-4 s^-1

giving a fundamental period of:

n_o^-1 = 6090 s (101.5 min)

with T_e set equal to 280K and g_d, .98K (10⁵ cm)^-1. The value for g, .95K (10⁵ cm)^-1, was read from the Pittsburgh sounding, which exhibited the most unstable lapse rate averaged through the column during the test period.

To begin to relate the hydrodynamical response within this macroscopic quantum domain, we introduce the fundamental frequency, or time ordering function, into the aforementioned expression for t, i.e., sin 2pn_ot. The values given in Table 1 detail the quantized signal originating at the perimeter of the rotating cluster of squalls, commencing on or about 1900 GMT. The initially hydrostatic, nonaccelerative atmosphere lying dormant to the north and south of Lake Erie thus receives three wave packets by 2325 GMT, each of which, upon passing a particular geographical point, excites a localized accelerative (nonhydrostatic) flow. Note that the wave period (50 min) is one-half n_o^-1, thus yielding a wave frequency of 2n_o.

The appropriate equation describing such “shallow-water” gravity waves traveling upon the inversion’s density anomaly is provided by Thompson (1961). The phase speed of these external-type of gravity waves

is equal to the mean potential energy given by g multiplied by the height to the base of the undisturbed inversion (3 km at the wave origin). The value of the term within the parentheses (.0312) is calculated from a density ratio formed between the average q within the underlying unstable atmosphere (q₁ = 280k) versus that of the stable layer (q₂ = 289k). The value of c, ±30.3 m s^-1 at the wave source, divided by 2n_o yields an initial wavelength of ±92 km. This initial wavelength is subsequently contracted to 80 km some 4 h later because the value of c decreases to 26 m s^-1 as the inversion slopes downward to 2 km. The combined information of changing phase speed and length of the waves emitted along the inversion allows us to construct Figure 4a.

During the passage of each individual wave packet, the local accumulation of atmospheric mass in the wave crests and depletion of mass by net divergence in the wave troughs imposes a periodic acceleration of air pacels beneath the inversion. The acceleration’s apparent cause is the resultant pressure gradients associated with the wave train. The surface pressure perturbations occurring beneath the limited atmospheric domain are shown in Figure 4b. The commensorate ascent causes air parcels to realize their diabatic potential, represented by their transit from (T_o) to
(T_c).

Before entering into a description of the role played by the Lorentz transformation in further delineating the limited atmospheric domain, some thoughts concerning the above underling of the words “apparent cause” seem in order. To say that the parcel ascends because of a local dominance of the pressure gradient term over the Coriolis force, in lieu of our extensive analysis of the quantized aspects of the dynamics, brings to mind the discussion by Bohm and Hiley (1975) regarding the relative independence of function existing as “only a special case of general and inseparable dependence”. We obviously concur with their judgement “that inseparable quantum interconnectedness of the whole universe is the fundamental reality and that relatively independent behaving parts are merely particular and contingent forms within this whole”. To paraphrase this important idea in light of the present case study, we would say that use of the traditional pressure gradient approach as the sole explanation for an accelerating atmosphere is to represent a self-limitation of the whole in a fashion which conceals certain functions (such as the relevance of quantum methodology applied to atmospheric dynamics) in order to highlight others. Such use of traditional dynamical explanation is not incorrect, only less encompassing in its account of universal phenomena. Perhaps the crucial line separating traditional explanation from that offered in the spirit of quantum and relativistic physics has to do with the former’s emphasis upon isolated structure versus the latter’s proper emphasis upon reciprocating function.

5. General Relativity and the Limited Atmospheric Domain

Alterations of the gravitational field affecting air parcels traveling within the limited atmospheric domain (Figure 1a) imply that relativistic physics is intimately tied to quantization of energy explained in terms of n_o. Furthermore, because of the abrupt change of parcel buoyancy across the inversion, we suspect that this thin membrane, acting as an effective wave guide, is the ideal place to elaborate upon connections between quantum and relativity theory. We, thus, introduce the Lorentz-Fitzgerald transformation into our work in order to explore the spatial limit distinguishing between the embedded and embedding domains.

From Erie (Pennsylvania) to Huntington (West Virginia), the difference in enthalpy plus geopotential energy (C_pT + gz) calculated upon an (284K) isentropic surface within the inversion was found to equal 0.7 x 10⁷ cm² s^-2. Dividing this by the north-south distance of 393 km orthogonal to the wave train (Figure 4a), we obtain an acceleration potential of 0.178 cm s^-2. If this value is assumed constant during the 4 h history of the first wave packet, then an external gravity wave commencing along the inversion at 1925 GMT would possess ageostrophic wind components which would increase from 0 to 25 m s^-1 by 2315 GMT. An exponential growth rate is indicated when we plug in the appropriate values for V_ageo(Table 2) and allow for a foreshortening of the wave length to 80 km (as is dictated by l = c/2n_o) using the formula:

A wave amplitude of more than 2 km is predicted at 2315 GMT, but since the inversion just to the south of Huntington was only 2 km above the surface terrain, the air parcels within the wave must have begun to “scrape bottom” in a manner analogous to shallow-water waves breaking upon a beach. The vector values of V_ageo do not enter into formula (26), but they do play a role when wave-packets are absorbed at critical layers aloft, i.e., the direction encoded in the wave-packet must match the direction of geostrophic flow for absorption to occur.

Upon calculating the acceleration of the inertial mass, manifest by the first wave packet as the increasing spin of air parcels in the vertical plane, we note from the Lorentz-Fitzgerald equation:

that the contracting inertial mass b becomes infinite where the initial mass (m_o) accelerates to a velocity (V_ageo) equal to the phase speed of the external gravity wave. It is here that the potential energy is entirely converted into kinetic energy. This mathematical specification of “infinity”, to be elaborated upon using the previously derived wave Eqs. (21a and 21b) and (22a and 22b), was first encountered in the formula for n_o (Eq. 19). The coefficient of t becomes negative where g > g_d : meteorologists describe this as a superadiabatic lapse rate. This extremely unstable case, which isolates where V_ageo equals c, yields a solution to Eq. (17) in terms of exponentials of time.

The formidable problem of an infinite term appearing within a differential equation is here resolved when we employ the coherent wave equations. These equations state that appearance of an infinite regress invokes the two imaginary components:

1. The first component () governs the first-order temporal exchange of information represented by a quantized horizontal component of spin being twisted orthogonal to the imaginary (horizontal) plane within the boundaries of the limited atmospheric domain. This information exchange is manifested as the appearance of a of relative vorticity.

2. The second component () governs the second-order temporal exchange of information between the embedded (limited atmospheric) domain and its embedding domain. This is manifest as a signal originating from collapse of the horizontal component of spin occuring at the breaking external gravity wave. This signal is identified with acceleration of a of spin within the embedding domain.

We use relative vorticity and (atmospheric) spin interchangeably. The most relevant point concerning the first- and second-order exchanges of information has to do with “Maxwell's demon”. That is, when an as-if-frictionless process is found to occur in the atmosphere, this is because moist diabatic processes insure that frictional losses are exactly compensated, such that the posentropic removal of velocity in the lower regime gets rewritten at the negentropic level of wave-packet absorption. This is precisely what J. C. Maxwell envisioned when he put his demon in control of a frictionless trapdoor bordering two gas reservoirs and set him about the task of selectively allowing only molecules (wave-packets in the present case) of given velocities to pass from one reservoir to the next.

The Huntington wind profile shown in Figure 5d, which was drawn from data accumulated during the release of a radiosonde at 2315 GMT, shows the resultant anomalies in the momentum field predicted by these wave equations. Two momentum “spikes” near the 2 and 4.5 km levels provide documentation that the two quantized exchanges of information have, indeed, occurred. Momentum shelves represent of relative vorticity established as narrow jets appearing in the horizontal plane. The 800 mb (2 km) jet acting as an accelerator of cold air from the western Ohio Valley, versus the diabatically warmed air over Lake Erie, is the mechanism by which the initial quantum signal, or subsystem-system temperature perturbation of 7°C, is fused into the entire limited atmospheric domain (Figure 6).

The partial erosion of an expected signature of two @ 25 m s^-1 momentum anomalies observed at 8 and 18 minutes into the radiosonde launch can perhaps be explained as insufficient data resolution. Also, in examining the Richardson number (Ri), or the ratio between the thermal and kinetic stabilities:

the sounding taken at Huntington confirms that two zones of critical Richardson numbers (£ 0.25) exist at both momentum spikes. Two layers of @ 540 m thickness appear at 2 and 4 km. Such critical values have been empirically found to be associated with Kelvin-Helmholtz, wave-induced turbulence, the frequency spectrum of which extends into the infrasound range (fractions of Hertz).

The means by which momentum is deposited at 4.5 km within the embedding domain is of interest because of its profound effect upon tropospheric, and then stratospheric, dynamics. The stipple in Figure 7a shows the close proximity of the upper momentum shelf to another inversion (or tropopause boundary) located by the uppermost, bold solid line. As ageostrophic momentum, depicted by the solid arrows, accumulates in the stippled area, the postulated folding of the tropopause transpires, indicated by the dashed bold line, caused by the ensuing accelerations. Figure 7b indicates that, twelve hours later, the folded tropopause boundary has “broken”, allowing stratospheric mass and momentum to flow downward along the newly formed frontal boundary (Figure 7c).

That this repeating, quantized sequence, originating from microscopic tropospheric physics, simply continues to be communicated from embedded to embedding domains is indicated by the deep vertical analyses of the of potential vorticity extending into the middle stratosphere (Figures 8a and 8b). This mathematical product of a system's vertical component of relative vorticity multiplied by the prevailing hydrostatic stability clearly exhibits the result of an exchange of information originating in the earth's lower atmosphere. The deformation of potential vorticity found near 30 km twelve hours after 0000 GMT 5 November is especially intriguing, for it occurs in a zone where microphysical stratospheric processes play a key role in the macroquantum dynamic. Whereas our discussion began within a region rich in the triatomic molecule H₂O, lack of data beyond the 30 km level prevents us from examining the quantum response, which would incorporate the triatomic molecule, O₃. Each of these molecules is the primary diabatic agent within their respective domain, i.e., the troposphere and the stratosphere. However, Figure 9a gives enticing evidence of yet another, larger spatial 7°C temperature anomaly occurring within this volume, containing values of the ozone mixing ratio ³10 mg g^-1.

To continue exploration of how all these dynamic regimes are coupled to the original fundamental frequency, n_o, we noted that the Huntington wind hodograph exhibited marked loops at 13, 38, and 44 min (Figure 9b) into the radiosonde ascent. We considered that the vertically propagating, acoustically-modified gravity waves generated these small inertial amplitude oscillations observed against the background geostrophic flow. Each wave can, thus, be represented by an ageostrophic vector rotating in the vertical plane at the tip of the mean geostrophic vector found at 3, 10, and 12 km within the embedding domain.

The traditional picture which thus emerges is akin to the undulating lower tropospheric inversion acting as a “piston” and modified acoustic wave source. The wave trace velocity

must, therefore, be a product of the final external gravity wavelength (80 km), multiplied by n_o. Jones and Houghton (1971) were able to show numerically that such vertically-propagating waves will deposit their momentum packets where the geostrophic flow equals V_t, 26 m s^-1 (52 kt). The postulated momentum shelf at 0000 GMT 5 November is shown in Figure 5d. This is noted as a significant anomaly which exists over and above the expected 52 kt flow at 4.5 km.

6. The Relativism of Absolute Limits

Starr (1959), so far as the authors have been able to determine, was the first to see that hydrodynamical waves are governed by E = mc². (In hindsight, this seems reasonable, since Maxwell a century earlier, in 1856, provided a theory of electromagnetic waves based upon hydrodynamical principles.) We would add, for purposes of practical application, that this total energy equation, formed by the product of an external gravity wave's gravitational and inertial mass, may be written:

Starr correctly concluded that the transport of kinetic energy (e) and potential energy (v) per wavelength,

where e_x and e_z are the contributions to the kinetic energy from the horizontal and vertical parcel motions comprising the wave, yields 2e_x. This is equivalent to subtracting the work done by the pressure forces, 2e_z, which, as we have already mentioned, contribute no component to the actual information exchanged within a limited atmospheric domain. To quote Starr (1959):

Of all this infinity of particles, none can have a velocity exceeding in magnitude the wave speed c. As a limiting case, only certain elements of measure zero can actually attain this critical speed, that is, those at the sharp crests of the waves. Furthermore, as the fluid elements approach this state of motion, they also approach a state of infinite contraction along the direction of wave propagation.

What we have arrived at is a more general concept than one attached solely to a limited atmospheric domain. Let us, therefore, define a Limited SpaceTime Domain (LSTD):

A Limited SpaceTime Domain is that limit, partition, or ordering function of space given by the ordering function assumed by time through a fundamental (“ground state”) frequency, n_o.

To further elaborate upon the universal nature we attribute to the physics of limited spacetime domains, let us list the first-order coherent wave equations alongside the analogous equations given by Maxwell:

where we have introduced the imaginary unit vector () to designate information exchange contained solely within the specified LSTD's. Eq. (32a) states that if all of the rotation within the geostrophic plane were absorbed at a vertical axis of spin, there would be a corresponding local change experienced in the ageostrophic velocity field, weighted by the coefficient +c^-1. This would effect the changeover from relative vorticity into . A parallel expression in Eq. (32b) states that if ageostrophic rotation were absorbed at a horizontal axis of spin, there would be a local change within the geostrophic velocity field, weighted by
-c^-1. This would effect the reverse crossover in relative vorticity components. Note that the ± signs for c are determined solely by the acceleration/deceleration of air parcels, respectively, with regard to the ageostrophic framework.

The first-order hydrodynamical theory (Eqs. 32a and 32b) leads to a conservation principle for the three spatial components of relative vorticity within a closed dynamical system. In testing Eq. (32a), we note that +59 x 10^-6 s^-1 units of macroscale, spin must have been absorbed over Lake Erie, if [1] c = 30 m s^-1, [2] the period of absorption of spin covers 4 h, and [3] the final resultant value of V_ageo equals 26 m s^-1. Eq. (32b) uses virtually the same values, except c = V_ageo = 26 m s^-1, and, hence, the re-emission of relative vorticity over the Ohio Valley is modulated to reach +69 x 10^-6 s^-1 units of spin.

The observed changes in patterns of the vertical component of relative vorticity exhibited in Figures 10a and 10b, as diagnosed upon the reference level 280K isentropic surface, do, indeed, indicate that the advection of cyclonic vorticity across the Great Lakes was interrupted by an absorption and re-distribution process. This process of 100% absorption-emission is, thus, a hydrodynamic analogue to blackbody physics as it is applied to thermodynamic problems.

A schematic diagram of the double helices resulting from the crossover from spin into of spin for individual air parcels within the LSTD is shown in Figure 11. The nearly horizontal tracks, for each parcel initially participating in the geostrophic macroscale flow, undergo progressively increased ageostrophic undulations as the parcels become part of the mesoscale wave phenomena in their approach toward the rotating convective cluster. When net spin in the horizontal plane is entirely cancelled upon reaching the squall's perimeter, the parcels become elements of a roll cloud. As viewed from the quantized hydrodynamical theory, this prominent feature of many rotating squalls producing tornadic activity represents the development of purely horizontal components of spin at the expense of macroscale, spin.

Eqs. (33a) and (33b) elaborate upon the nature of discharge between magnetic and electric field intensities, just as Eqs. (32a) and (32b) describe how energy-momentum may be exchanged between the geostrophic and ageostrophic velocity fields. Specifically, if curl is absorbed at a mathematical singularity, there will result a local change with respect to time of , weighted by +c^-1. Likewise, if curl is absorbed at a mathematical singularity, there will result a local change with respect to time of , weighted by -c^-1. The units of c in both sets of equations are those of velocity, where the former c is determined by the units of acceleration divided by rotation. As suggested by the parallelism, the “atmospheric” c is the absolute limiting phase velocity of those waves which accomplish the perfectly efficient transfer of energy-momentum within a LSTD. In the electromagnetic domain, c is the velocity of light which permits the changeover between units of electrodynamics and electrostatics. In the atmospheric domain, c_atmos @ c_light x 10^-7, the limiting velocity governs the crossover between non-hydrostatic and hydrostatic physics.

7. An Initial Look at the Superconductant Role of Nonequilibrium Negentropic Processes

If we now describe the divergence of the two vector fields set to zero, this mathematically closes each field, or bounds it in such a way as to prevent an inexplicable escape of energy-momentum from the limited atmospheric domain:

The set of four equations (32a and 32b; 34a and 34b) which we have derived for studying a superconductant atmospheric domain exhibiting superfluid properties depends upon the supposed absence of external friction. This is an unreasonable condition, given that the lower boundary of the limited atmospheric domain is the surface of the earth. Likewise, Maxwell's set of mathematically similar expressions, including those for the divergence of the magnetic and electric field intensities, set equal to zero:

are valid for situations other than those in which there is an absence of conductors and free space charges.

The idea that the limited atmospheric domain bounded by the earth's surface behaves as if it were shielded from external friction is a puzzling paradox arising from our mathematical theory which needs to be sorted out. The solution to this dilemma should provide investigators with clues as to how electromagnetic superconduction can occur at temperatures far above absolute zero.

In order to isolate this problem, we provide a sample in Table 3 of the drag coefficients (C_d) calculated using the formula provided by Jensen (1973):

The drag coefficient, a variable commonly used to proportion the transport of energy through the planetary boundary layer, is most dependent upon the varying depth to the base of the inversion (H), the angle between the observed and geostrophic wind vectors (SIN d), and the inverse square of the observed wind at the surface. Other variables include the magnitude of the geostrophic wind and f, the Coriolis parameter (~10^-4 s^-1). After a departing external gravity wave crest passes overhead (approaching trough), the supergeostrophic (accelerative) flow responding to the local pressure perturbation organizes an intense flux of sensible and latent heat into the limited atmospheric domain. A nil or weak counter flux occurs after the wave trough passes individual stations.

The two orders of magnitude variation in the drag coefficient allows the moist primitive equation model to “feel” the real-world influences of microeddies which fall within the numerical grid spacing. Although these microeddies within the planetary boundary layer represent a localized positive viscous and entropic process, the view of their role from the perspective of the entire limited atmospheric domain is that of a negentropic (negative viscous) process. Eq. (32b) describes the simultaneous negentropic dynamic which serves to supply a diabatic potential to the domain. [[COS 2pn_ot, introduced into Eq. (17), records this negentropic aspect of the external gravity wave phenomena.]] Thus, as the largescale wave train alternately excites and then suppresses the surface flux of diabatic energy, quantized packets of “free energy” are introduced into the limited atmospheric domain.

The external gravity wave train then goes on to organize the subsequent release of diabatic potential within the limited domain. By thus synchronizing the introduction of energy into the LSTD through its lower boundary, the sensible heat realized during the production of precipitation within the storm environment serves to sustain and amplify the planetary boundary layer pressure perturbation associated with the external gravity wave train. This, in turn, manufactures momentum at the larger lengthscale which exactly compensates for the loss the system suffers through external friction. THE INVOLVED “EXACT COMPENSATION” IS THE NECESSARY AND SUFFICIENT CONDITION FOR SUPERCONDUCTANCE of hydrothermodynamic properties. It is but a small leap to realization that charge is not the only property capable of superefficient conductance. Solitons and superfluidity are, indeed, other such cases.

To examine the exchange of information which exists between LSTDs, we will consider the second order wave equations. We are thus encouraged to write the Maxwellian analogue to Eqs. (37a) and (37b), as listed below:

A more detailed, quantified account of the information exchange between embedding and embedded domains will be given in section 9, after we have firmly established the quantum aspects of the external gravity wave phenomena.

In summarizing these approaches to superconductant physics, we have found that the relevant atmospheric equations have their exact analogue in Maxwell's equations when we employ two aspects of time ordering. This has been accomplished by separating the dynamics of the atmosphere into two vectorial fields described by the geostrophic and ageostrophic velocities. The geostrophic field contains vortices measured within an incompressible (isentropic) frame of reference which is alternately charged and discharged of energy-momentum by the ageostrophic field of motion. This compares favorably with Maxwell's mathematical description of an idealized incompressible fluid, which he believed to be subject to a species of (internal) friction invoked to explain the relationship between electric and magnetic fields.

BOTH EQUATION SETS DELETE THE MASS ASPECT OF THE DYNAMICS BECAUSE THEY SEEK ONLY TO DESCRIBE THE INFORMATION TRANSMITTED, THUS ALLOWING ACTIVE TIME TO REPLACE THE TRADITIONAL WORK TERM ACCOUNTING FOR THE MEANS OF TRANSMISSION. Hence:

Time dictates accelerations in the relativistic-quantum approach, thus yielding an equivalence principle which equates the physics of acoustic waves to the physics of gravity and electromagnetic waves.

One is led to the conclusion that an explanation of the synchronized cascade of quantum potential below the subsystem (molecular) level would employ the Maxwellian equation sets to explain the unusually active thunderstorm phenomena observed during the test period. This should be developed during future investigations of superconductant, limited atmospheric domains.

8. Defining the Quantum State within the Limited Atmospheric Domain

If one considers the multiplicity of quanta which we have now identified from a single fundamental frequency (i.e., the external gravity wave train, the vertically-propagating internal gravity waves, the microeddy introduction of “free energy” packets, et cetera), then an intriguing picture emerges which will require a reinterpretation of Schrödinger's wave equation. A given fundamental frequency of an oscillating parcel represents a state (i.e., relative-state, in the full Everett-Wheeler-Graham sense) of interaction between the limited atmospheric domain and a given level of its embedding hierarchy. All the whole integer packets of quantized energy and angular momentum are quanta arising from the superposed subsystem-system-supersystem composite. And the description of the total configuration of oscillating parcels, each represented by a given eigenvalue having real and imaginary components, is a deterministic description of the result of all possible interactions of the LSTD with its embedded subsystems and embedding supersystems.

Likewise, the complex nonlinear wave interaction, both within and outside the embedded domain, must be represented by superposed eigenfunctions having real and imaginary components. Each process carried to its limit generates sets of quanta which serve to interconnnect all involved domains. This interaction is currently described as if it were a probabilistic wave function tied to a single (linear) view of time. But what if Schrödinger's equation were re-written, as we have demonstrated with the equation set of Maxwell, to include two ordering functions of time (one being nonlinear)? Would we then begin holding the entirety of such multivalued, complex, mathematical values and functions in view so as to encompass the vistas offered by a hierarchical-holographic, relativistic-quantum theory?

a. Planck's Law

To establish validity of such an approach, as well as to put the equivalence principle for gravitational, acoustic, and electromagnetic waves on a firmer theoretical footing, we will test our conceps by reinterpreting Planck's Law for the limited atmospheric domain.

In combining Wien's and Rayleigh-Jean's Radiation Laws, Planck effectively described the quantized aspect of radiation communicating information between long and short wavelengths within the electromagnetic spectrum. In the atmosphere, severe local storms arise when the short wavelengths (acoustic gravity waves) are excited by a cascade of angular momentum and energy released by the unstable longwave train (³ 1000 km). Thus, the longwave train, upon being subjected to a critical net radiation exchange, triggers the flow of information across the spectral gap.

In the test case, the resultant change of entropy

was determined by the slope of a constant entropy surface multiplied by C _p. The oscillator, having temperature T and entropy S, plus a fundamental frequency n_o, determines h* in the atmosphere for this particular limited spacetime domain:

Hence,

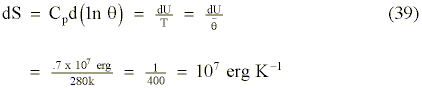
Knowing h*, the fundamental period for spontaneous localization at the subsystem (molecular) level becomes h*/C_pT or 7.5 s (see Baracca, et al., 1975). [[The inverse of h*/C_pT yields .13 Hz, the theorized fundamental frequency of the vertically-propagating infrasound. These acoustic waves amplify exponentially as they propagate into the upper atmosphere, because of the decreasing density with respect to height. This gives insight into the means by which tropospheric dynamics are coupled to the ionesphere by the quantum signal. It also provides quantitative guidance to evaluation of the risks ground-based transmitters, high-energy lasers, and man-made hot auroras pose to processes involved in ozone metabolism.]]

The ratio of the Laplacian of entropy relative to the “ground state”, between the atmospheric shortwave (analogue to Wien's Law),

and longwave class (analogue to Rayleigh-Jean's Law),

is simply (H*n)^-1, or (.7 x 10⁷ erg)^-1. Eqs. (41a) and (41b) contain the value for the energy of the radiant field (or “ground state” energy),

where h*n/C_pT gives the aforementioned ratio of 1:400. (Of course, the value for U is also given by multiplied by C_p.) The energy density of the oscillator,

where c is taken to be 30 m s^-1 and n = 2n_o as before, may be given in terms of the more customary dimensions of an atmospheric “parcel”, i.e., a cubic kilometer (10¹⁵ cm³ = 1 km³): Un = 280 x 10⁷ erg km^-3 s^-1. Finally, the radiation intensity at a specific frequency,

when factored from its initial units involving an “instantaneous” vorticity (angular momentum) production (s^-2), may be recognized as containing the relative vorticity communicated to the Ohio Valley by the ensemble of air parcels comprising the first wave packet.

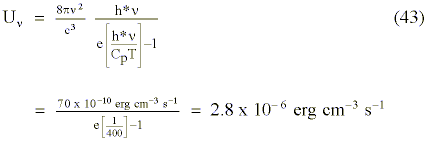
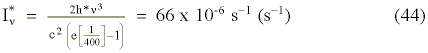
b. The Relativistic-Quantum Definition of Temperature

We have now come full circle to centering our attention once again upon the temperature field defining a macroquantum state. Beginning with Maxwell's kinetic theory of a gas, temperature has been defined in terms of a statistical average involving the ensemble of molecules moving at varying velocities. Nothing specific could be said with regard to the kinetic energy of a specific molecule located at a spatial coordinate. Or, knowing the momentum of the molecule, its spatial position remained elusive. Maxwell, did, however, conceive of his famous “demon” in imagining an alternate view of reality which bears a remarkable resemblance to the relativistic-quantum approach to dynamics:

The macroquantum description of a superconductant domain treats TEMPERATURE AS A MAP OF DIFFERENTIAL SPIN whose temporal configuration is precisely described.

Let us investigate this idea further, by returning to Planck's description of the energy of the radiant field.

We recognise that the ratio of h* n/C_p replaces hn/kT in the electromagnetic domain, where k = 1.38 x 10¹⁶ erg k^-1. How does the temperature field, diagnosed upon the reference level isentropic surface (designated by ), relate to vertical and horizontal components of spin? If the macroscale of relative vorticity of the limited domain goes to zero, i.e., , then the quantized packets of of vorticity may be calculated using the acceleration potential, . Recall that such communication serves to inform higher frequency phenomena which are capable of altering the limited domain's temperature field. Realization of the acceleration potential, in turn, taps a “free energy” (diabatic potential) which restores to the limited atmospheric domain a redistributed of relative vorticity, while also altering the temperature field upon the constant entropy reference level.

That vertical variation of temperature is closely tied to vertical acceleration has already been thoroughly examined in Eqs. (1) and (2). But what of the horizontal variation of temperature being an alternative for interpreting air parcel inertial stability in the quasi-horizontal insentropic plane? To illustrate this idea, we write an equivalent expression to Eq. (1), only we now replace g with the Coriolis force (fV_geo), while also replacing the vertical pressure gradient (which could be expressed as ¶P/¶r_earth) with the pressure gradient determined along a quasi-horizontal isentropic surface:

In direct analogue to the hydrostatic approximation, we then establish the definition of a balanced thermal field dictated by the Coriolis force by once again invoking the equation of state differentiated with regard to a radius leading to a macroscopic spin
() center:

Plugging the result into (45), we obtain

This defines the lapse rate associated with an isentropic surface's relative vorticity center. To show that this quasi-horizontal, adiabatic, or “geostrophic” lapse rate does agree with typical meteorological fields, we provide an example using [1] f set to 10^-4 s^-1, [2] V_geo set to 20 m s^-1, and [3] R set to 2.87 x 10⁶ cm² s^-2 g^-1 K^-1. The resultant value applied at latitude 45° within a typical atmospheric scalelength equals -3.5°C (500 km)^-1.

In the present case study, the variation in the 280 K surface's temperature field from Lake Erie to the Ohio Valley was more than twice the appropriate g_geo [-7.0°C (400 km)^-1]. Subtracting out the geostrophic lapse rate, the ageostrophic lapse rate of @-3.5°C (400 km)^-1 gives an ageostrophic component of 25 m s^-1 using Eq. (48):

This excessive lapse rate thus records the inertial instability of the fluid, which we have documented in terms of a significant ageostrophic flow. This is exactly analogous to a vertical inertial instability arising wherever the vertical pressure gradient exceeds the gravitational damping capacity, only here it is the Coriolis force which is unable to “balance” the quasi-horizontal pressure gradient. Figures 12a and 12b show the influence that the resultant accelerations have had in changing the pressure field upon an isentropic surface located beneath the inversion. Eq. (49) states that such perturbed pressure fields may also be used to calculate V_ageo, once we eliminate the geostrophic pressure gradient:

The equations derived in this section, part b, prove useful in defining the initial conditions for programming the coherent wave equation sets for computer simulation (as has extensively been done with many historical data sets, in association with Dr. Michael Kaplan of the NASA-Langley Research Center, in course of performing multiple nested-grid computer simulations of tornado genesis with the full-spectrum, primitive equation model -- which proved capable of “predicting” tornado outbreaks, on basis of the historical data sets, up to 12 hours in advance on a 1 km grid).

Although we have illustrated a new application of temperature fields using data from the atmospheric limited (spacetime) domain, one should not overlook the profound effect that temporal ordering has in simplying the N-body problem and questions of indeterminacy. A supposed singular (selfsame) universe, built upon spatial referencing, wherein each entity has simple-identity and is localizable does, indeed, exhibit indeterminacy by virtue of the vast array of hidden influences communicated to and from limited spacetime domains which have not been distinguished one from another. In contrast, the notion of a “multi-sheeted” or “laminated” universe composed of multiple superposed (interfused) “universes” i.e., LSTDs, built upon the ordering functions of time, promises to extend our understanding, by giving new meaning to concepts like temperature.

9. On the Relativistic-Quantum Domain and Nonequilibrium Thermodynamics

We have yet to explore exactly how the surface flux of moisture into our regime, followed by a full realization of this diabatic potential, is able to return the limited atmospheric domain to a state of equilibrium. To examine the involved concepts in depth, we shall be required to describe a self-inductant circularity arising between the embedded and embedding domains.

To enter into this description, we will require some of the computer simulation products provided by the multiscale primitive equation (PE) model of tornado genesis and onset of other severe local storms, first mentioned in Section 4. The nested-grid approach, now undergoing further development by Dr. Kaplan, has proven invaluable to our understanding of the complexities of the severe storm environment. In the numerical procedure, we “step-down” eight times by repeatedly halving the initial hydrostatic gridlength to eventually simulate the nonhydrostatic physics requiring gridlengths of less than 1 km (Paine, et al., 1978).

However, we quickly learned from these numerical simulations that the objectively-analyzed computer graphics displaying traditional field variables literally overwhelm one when dealing with such a rapidly changing, multivalued dynamic. The multiple values of the primitive variables (and their associated mathematical terms in both the diagnostic and prognostic equation sets) arise because, at any given instant of time, there exists an ensemble of wave phenomena. Each frequency window in the numerical prediction, thus, displays the simultaneous solutions to the nonlinear differential equations generated in finite time steps varying from 60 s to 1s.

When we first began generating such simulations, our goal was to develop new display techniques which would effectively synthesize these multivalued solutions in a readily discernible fashion. Figure 13 shows the predicted q_E-surface which participates in both the perimeter of the rotating cluster of squalls as well as the low-level jet structure. (Remember that the value of 287 K is an average equivalent potential temperature within this LSTD, which exhibited a q_E range from 281 to 293 K.) This surface is effectively a visual representation of the total static energy field, C_pT + gz + Lw_s , where L is the latent heating constant and w_s is the saturation mixing ratio for water vapor. The highly contorted topography is shown at 5 h into the PE simulation of the Lake Erie storm. Figure 14 shows the accompanying predicted vertical motions at the hydrostatic, 32 km gridlength, along with the observed convection. The view is relative to a slice taken through the 291 K q_E-surface, looking along the 875 mb level, which is located well below the inversion within the severe storm.

The spiraling bold lines, in Figure 13, represent quasi-Lagrangian solutions to the energy balance equation computed from the PE variables for three individual air parcels (see Appendix C). These parcels:

1. first participate in the intense, three-dimensional convergence and acceleration of moisture-laden air drawn upwards into the rotating squalls;

2. upon realizing their positive diabatic potential, are ejected from the convective canopy or anvil formed where the buoyant updraft rapidly decelerates within the inversion;

3. then descend as each experiences a “negative” diabatic potential (T_p returns to T_o from T_c) as evaporational cooling ensures.

If the atmospheric surface pressure patterns were explained by the lower tropospheric velocity and mass convergence/divergence, then we would expect the pressure field beneath the convective squalls to be anomalously high, while the strong subsidence region ahead of the squalls would have an anomalously low pressure. Instead, Figure 2 and Figure 4b show the opposite observsed pressure anomalies.

Counteracting, by computer simulation, mass and velocity divergence/conver-gence, on the order of ±10^-4 s^-1 near 4 km, associated with the newly established momentum shelf, was found to effectively offset the adiabatic convergence/divergence within the planetary boundary layer. Several investigators have concluded that such mass divergence aloft triggers both the lower tropospheric convective storm as well as any associated low pressure anomally. However, our data does not support this scenario, which, in the present instance, at least, saw the momentum shelf and subsequent mass and velocity divergence on the order of 10^-4 s^-1 develop after the onset of lower tropospheric convection. Either way, the near-zero net integrated divergence within the atmospheric colums in question suggests that the adiabatic motion field does not exert a direct control upon the pressure anomalies observed at the surface within the severe storm environment.

We speculate that the explanation for the mesolow and mesohigh, respectively, lie within an evaluation of the local change in density realized by intense diabatic (condensational) heating, followed by equally intense evaporational cooling. The latter would occur as some of the ejected parcels carried their precipitation load into the storm's surrounding dry environment. This often appears in radar and visual studies of intense convective phenomena as a precipitation shaft, which is literally flung into the dry-subsiding air surrounding the storm.

The ejected, precipitation-laden air would counterbalance the mesolow's pressure decrease within the (embedded) LSTD by building the opposite pressure anomally in the accompanying embedding domain. The ±7 mb observed anomalies thus reflect mass conservation between the domains. Appendix C further describes the offsetting entropy changes which result as the static and kinetic energy of the parcels adjust to effect a net conservation of energy between the two domains.

However, the most compelling evidence for this speculation comes from solving the total energy equation (E = mc²) describing the mass to energy transformations within the LSTD. In the present study, 4.6 mg g^-1 of condensate (w_s) was found to yield -.65 x 10⁷ erg g^-1, when evaluated within the diabatic energy term, -Lw_s 1n q_E (Hess, 1959). This offsets V²_ageo, the horizontal kinetic energy (2e_x = +.65 x 10⁷ erg g^-1) realized within the amplifying external gravity wave, whose origin was traced to the perimeter of the squalls. This indicates that the acceleration potential is re-distributed throughout the limited spacetime domain, thus adding up to a net unchanging velocity field and return to the nonaccelerative state, once the quantum signal has itself been radiated from the domain. We conclude:

The alteration in the geopotential field contributed by the realization of the full (positive) diabatic potential generates an inertial mass characterized by a CRITICAL kinetic energy. This is exactly counterbalanced by the diabatic energy released within the quantum signal generator (convective squalls). The involved “exact counterbalancing” is characteristic of superconductant transport of hydrothermodynamic properties of a system.

A second-order, total energy equation is necessary for description of the two coupled relativistic-quantum domains. Einstein's classic expression is, therefore, re-written with primes to indicate the “connective-mass-energy” tying the embedded and embedding domains together by means of the limiting acceleration:

We interpret m', with units of g (s^-2)^-1, to identify the total time tendency of divergence and vorticity exchanged between the embedded-embedding domains per unit of exchanged mass condensate. Eq. 50 states a conservation principle exhibited between domains:

The development of divergence and (anticyclonic) relative vorticity accomplished by realization of the full (negative) diabatic potential within the embedding domain supplies the necessary decelerations for re-ordering the embedded domain.

To evaluate Eq. (50), we note from Eq. (37a) that a value of approximately -2 cm s^-2 for c' is computed

using the known absorption of relative vorticity supplied by Eq. (32a). We further assume from the diabatic trajectory computations that this was accomplished by the acceleration of parcels to [+30 m s^-1 (7200 s)^-1] within the mesolow, followed by a deceleration [-30 m s^-1 (7200 s)^-1] within the mesohigh. The temporal Lapacian thus employs a denominator of (7200 s)² centered as a finite difference upon the value of = c = 30 m s^-1. We now approximate m', knowing that a change from -10^-4 s^-1 (mesolow) to +10^-4 s^-1 (mesohigh) units of divergence was observed within the planetary boundary layer. We find the connective-energy

is released by the transformation accomplished by the limiting acceleration acting upon the connective-mass (condensate). Recall that this more than ten-fold increase in energy, which is required to re-order the original limited spacetime domain, is derived from microphysical processes occurring at the sub-parcel (molecular) level, and, thus, is certainly involved in ozone metabolism. We also note that E' is approximately one-tenth of the total enthalpy characterizing the limited atmospheric domain.

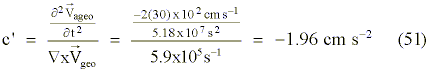
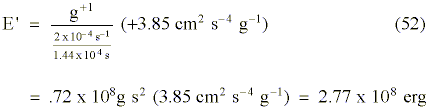
This gives us important insight into the counterposed roles of positive-negative entropic and viscous processes operating within the embedded-embedding domains. The adiabatic description of this dynamic predicts a continuing increase of pressure beneath the squalls (mass densification crisis), while similarly decreasing the pressure preceding the squalls. In contrast, the nonlinear or cyclic influence of diabatic physics, outlined here, predicts a prolonged pressure perturbation of the reverse sign. This latter result is in agreement with the observed data.

By prolonging the “bubble” of high pressure which wraps around the mesolow, the embedded domain is forced to entrain air from the embedded domain. This, in turn, terminates the role of diabatic processes operating within both domains. The parcel response to the opposing pressure gradients at the surface and inversion boundaries subsequently causes a mixing of the properties distinguishing the two domains to rid each LSTD of their respective static and kinetic energy anomalies. This mixing is represented in Figure 13 by the dashed extensions to the subsiding parcels which are shown to be re-entrained into the convective storm. With the renewed ascent of the parcels, the opposing (cancelling) diabatic signal enters into the convective storm as a stream of relatively cold, dry air.

The involved torus-type configuration of the flow field challenges the more limited view of information processing offered by purely adiabatic physics in describing the limited atmospheric domain:

1. An undifferentiated view of the embedded and embedding domains describes an emerging crisis of information densification
   (® ¥ in the atmospheric domain) brought on by the continuing pressure amplification dictated by net, three-dimensional mass and velocity convergence within a limited spacetime domain.

2. In contrast, the inclusion of diabatic physics states that the information densification will proceed just so far, then in a recycling sequence linking the embedded-embedding domains, the LSTD communicates the quantum signal as it “dismantles” the original radiation source. The domain thus evolves to a new reference state, ready to receive a new information exchange input.

The transmutation of connective-mass and -energy between the embedded-embedding domains includes the second-order time function of mass condensate-evaporate. Full realization of the positive and negative diabatic potential thus provides the necessary acceleration-deceleration to return the embedded domain to a state of order. This description of a limited spacetime domain, having the ability to self-inform while simultaneously returning from its CRITICAL state to a new reference state, appears to support the notion of “self-reference” described by Wheeler in Isham, et al., (1975). Starr (1959) extensively documented the importance of negative viscosity operating in dynamic systems, dynamic systems such as that described above. Prigogine (1971) and Prigogine, et al., (1972) have written extensively upon nonequilibrium thermodynamics applied to both hydrodynamic processes and biological systems. The self-referential aspects of the above described superconductant transport of hydrothermodynamic properties, involving negative viscosity, adds a multivalued dimension to the prevailing account of nonequilibrium phase transitions. Paine and Pensinger (1978) have, on basis of consideration of this multivalued dimension, applied the present mathematical model, combining a re-formulation of nonequilibrium thermodynamics as well as quantum nonlocality, in proposing a superconductant dynamic description of helix-coil transitions of the DNA molecule. This description portrays each nucleotide pair as being associated with a propagating COHERENT wave phenomenon, which communicates genetic information to cellular phase boundaries.

Finally, we observe that the sophisticated interplay between the lattice-like structure of the inversion and the COHERENT individual acoustically-modified gravity-wave-mode packets (atmospheric analogue of phonons), discussed above, adds considerable detail to the explanations offered by Bardeen, Cooper, and Schreiffer (1957) in describing superconductant phenomena. We might also note that the idea of a “macroquantum state free energy”, as introduced here, is especially interesting in this context when one considers that, on the subsystem or molecular level, Gibbs free energy is necessarily introduced into the microphysical explanation of snowflake growth from an embryonic nucleus. Time ordering, through CRITICAL and COHERENT processes, appears fundamental to form generation across scale levels.

10. Summary and Conclusions

Our model of a superconductant atmospheric limited spacetime domain may be summarized in verbal fashion: In the nonaccelerative (stable) state, the angular momentum of the domain remains at a constant entropy state tied to the fundamental frequency of an elemental oscillator, n_o. A quantum signal (change of parcel periodicity) and entropy increase originate when the system is subjected to a critical radiation exchange. The subsequent accelerations bring the system and its embedded domain(s) to a critical temperature, which we have alligned with a realization of the diabatic potential. The total energy density field then returns to the ground state -- defined by the domain's angular momentum once again having become aligned within the reference level of constant entropy -- as the quantum signal is radiated to the embedding domain(s). Section 9 elaborated upon how disorder generates order (decrease of entropy and viscosity): i.e., positive entropic and viscous processes introduce quanta of free energy from the embedding domain to accomplish the work necessary to return the embedded domain to its nonaccelerative state.

Eq. (17) has traditionally been written in terms of z, a reference height, in place of t. We have, essentially, substituted a reference “level” in time, in lieu of the traditional reference level in space. The “position” in time, or the characteristic “fine-structure differential interval” of periodicity in movement between T_o « T_c, becomes the medium of information transfer. This is to say that a hydrothermodynamic temporal-structure property, rather than a spatially constituted hydrothermodynamic entity, is exchanged in a superconductant fashion within and between limited atmospheric spacetime domains. The rate of change of this “position” in time constitutes the information transmitted within the domain [time rate as quantum-relativistic information unit], while the acceleration-deceleration of this “position” in time constitutes the information which flows between the embedded and embedding domain(s) [acceleration-deceleration of time rate as second-order quantum-relativistic information unit].

This mathematical model is ideally suited to dynamical studies in the areas of severe storm genesis, solar-terrestrial interaction, climate simulation, and weather modification. Significant statistical correlations in these areas of study may be tested using this analytic model and the data generated by increasing expertise in remote sensing of planetary atmospheres. We also note that a new standard for judging numerical model performance, as well as resolving boundary fluxes, both observed and predicted, is available by employing this relativistic-quantum methodology.

-Acknowledgments-

Three individuals, while the first author pursued his M.S. at the Pennsylvania State University, contributed in unexpected ways to the concepts formulated within this paper. Anton Chaplin, a co-graduate student enrolled in Dr. John Dutton's radiation course, did a term project in which he mathematically referenced an air parcel to a temporal, as opposed to a spatial, level. The last decade for the first author has been spent in pursuit of the implations of this statement. Dr. Edwin Danielsen did pioneering work in isentropic theory and analysis which has inspired a long list of students in the atmospheric sciences. Dr. Paine's own students at Cornell University have provided the necessary forum for discussing and testing these concepts. John Zack, James Moore, Robert Posner, Nathaniel Tetrick, and Eric Scace pursued various aspects of these topics as part of their own graduate research. Data gathering and analysis were performed with the aid of NSF GA-35250 and NASA-1365 grants at Cornell University.

12. References

Baracca, A., D. J. Bohm, B. J. Hiley, and A. E. G. Stuart, 1975: “On some new notions concerning locality and non-locality in the quantum theory”. IL Nuovo Cimento, 28, 445-465.

Bardeen, J., L. N. Cooper, and J. R. Schreiffer, 1957: “Theory of superconductivity”. Physical Rev., 5, 1175-1204.

Bohm, D. J. and B. J. Hiley, 1975: “On the intuitive understanding of nonlocality as implied by quantum theory”. Found. Phys., 5, 93-109.

Glansdorff, G. and I. Prigogine, 1971: Thermodynamic Theory of Structure, Stability, and Fluctuations. New York, Wiley.

Hess, S. L., 1959: Introduction to Theoretical Meteorolgy. New York, Holt, Rienhart and Winston, 54-56.

Isham, C. J., R. Penrose and D. W. Sciama (Eds.), 1975: Quantum Gravity. Oxford, Clarendon Press.

Jensen, J. V., 1973: “Synoptically-derived values of the drag coefficient”. M.S. Thesis, Univ. of Waterloo, Waterloo, Ontario, 66 pp.

Jones, W. L. and D. Houghton, 1971: “The coupling of momentum between internal gravity waves and mean flow -- a numerical study”. J. Atmos. Sci., 28, 604-608.

Kaplan, M. L. and D. A. Paine, 1973: “A 32-km moist primitive equation model providing for scale interaction”. J. Atmos. Sci., 30, 213-222.

_____ and _____, 1972: “A macroscale-mesoscale numerical model of intense baroclinic development”. J. Appl. Meteor., 8, 1224-1235.

Paine, D. A. and W. L. Pensinger, 1978: “A dynamical theory describing superconductant DNA”. In press: Intl. J. of Quantum Chem.

_____, J. W. Zack and M. L. Kaplan, 1978: “The cascade concept of severe local storm generation: horizontal convergence development”. Paper submitted to Mo. Wea. Rev.

Prigogine, I., G. Nicolis and A. Babloyantz, 1972: “Thermodynamics of evolution”. Physics Today, 25, 23-28.

Reiter, E. R., 1972: Atmospheric Transport Processes. Part 3: Hydrodynamic Tracers. Atomic Energy Commision, Critical Review Series, 212 pp.

Sanders, F. B. and D. A. Olson, 1967: “The release of latent heat of condensation in a simple precipitation forecast model”. J. Appl. Meteor., 6, 229-236.

Starr, V. P., 1959: “The hydrodynamical analogy to E = mc²”. Tellus, 9, 135-138.

_____, 1968: Physics of Negative Viscosity Phenomena. New York, McGraw-Hill, 256 pp.

Thompson, P.D., 1961: Numerical Weather Analysis and Prediction. New York, Macmillan, 170 pp.

[[THIS PAPER IS STILL UNDER CONSTRUCTION. CURRENT REFLECTIONS ARE YET TO COME.]]

---

Return to:

•Top

•The Ithaca Papers -- Abstracts

•Home page